BQ#7: Unit V: Deriving the Difference Quotient

How do you derive the difference quotient?

• To answer this question, we must first look at a couple of formulas and remember that we are basically going from step to step in order to achieve this end result. 

(http://images.tutorvista.com/cms/images/39/difference-quotient-formula.png)

         Really the only formula we will be using in order to get to the difference quotient is the slope formula because we need to find the slope of a line that goes through two points on the function graph called the secant line.

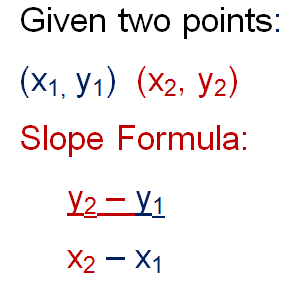(http://www.algebra-class.com/image-files/slope-formula-2.gif)

            But for now lets look at this picture and break it down to see how we get the points we need...

(http://cis.stvincent.edu/carlsond/ma109/DifferenceQuotient_images/IMG0470.JPG)

• On a graph of a function, assuming we don't have units labelled and all, we have no idea of knowing the exact value of those points we need. However, we can name the distance on the x-axis with a certain variable named x. Using this, we can add on this value of x with another variable that we will call h in order to get the distance to our second point on the x-axis. To get the necessary y-values, we just find the function of the x-values like we normally would with a function graph. Since we don't know the actual values, we leave both as f(x+h) and f(x). 

• The secant line is represented by these two points and now all we have to do is plug it into our slope formula to get our difference quotient. Remember that our first point is ( x, f(x)) and our second is (x+h, f(x+h)). Look back at the slope formula if you get lost. 

• Still confused? This video gives a good explanation on the entire process if you need more help.

References

http://images.tutorvista.com/cms/images/39/difference-quotient-formula.png

http://www.algebra-class.com/image-files/slope-formula-2.gif

http://cis.stvincent.edu/carlsond/ma109/DifferenceQuotient_images/IMG0470.JPG

https://www.youtube.com/watch?v=XA0fZh8cXV8